
Have our fourth term, we have the term minus one right there, the fourth term we have a three, the third term we have a two, the second term we have a one. So if you see the pattern here, when our term, when we Three times from the 15, so minus three times six. What's the fourth term? This is 15 minus, we're subtracting the six Now what's happening here? This is 15, this is 15 minus two times negative six, or sorry minus two times six, minus two times six. One times negative six, either way, we're What's the second term? This is 15, it's just we just subtracted six once, or you could say minus one times six.
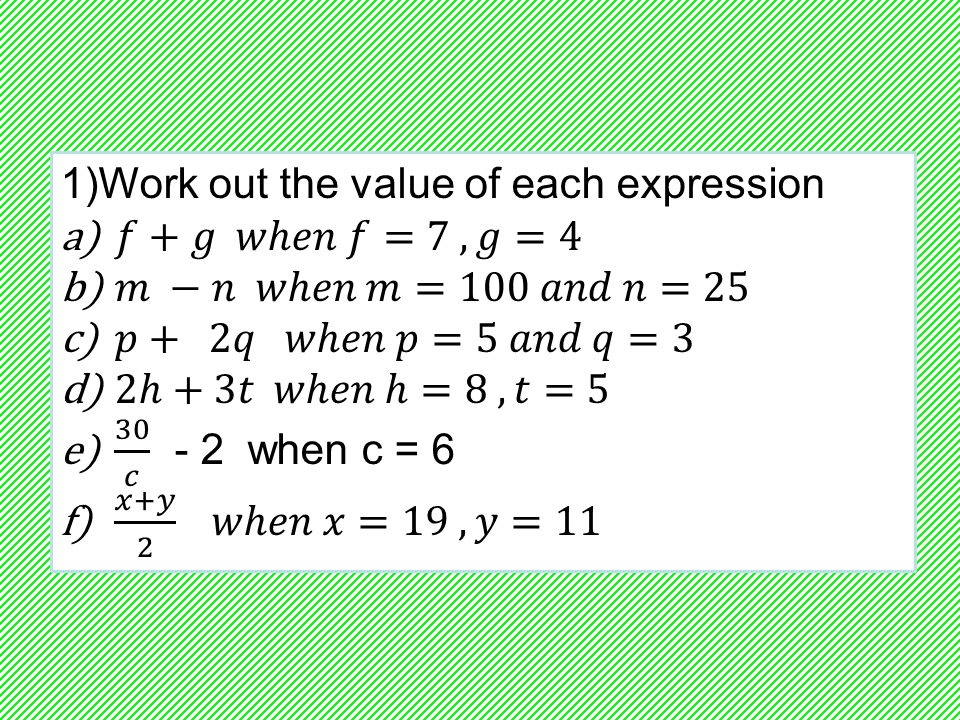
Write it better this way, minus zero times six. So you could say this is 15 minus six times or let me Subtract six at all, or you could say you Let me write this down just so, notice when your first term, you have 15 and you don't So whatever term you're looking at, you subtract six one less In the fourth term, you subtract six three times. So in the third term, you subtract a six twice. The third term is going to be 12 minus from the first term, or six subtracted twice. So the second term is going to be six less than the first term. So it looks like every term, you subtract six. Three to negative three, well we, we subtracted six again. Then to go from nine to three, well we subtracted six again. So we went down by six, we subtracted six. Think about just how much the numbers changed by. Term to the second term, what happened? 15 to nine, looks like So let's see what's happening here, if we can discern some type of pattern.

Out what the 100th term of this sequence is going to be. And they wanna ask, they want us to figure And then our fourth term, our fourth term is negative three. I'm just really copying this down, but I'm making sure we associate So if we have the term, just so we have things straight, and then we have the value, and then we have the value of the term. Asked what is the value of the 100th term in this sequence, and the first term is 15, then nine, then three, then negative three.


 0 kommentar(er)
0 kommentar(er)
